[Uni Tübingen] - [Mat.-Nat. Fakultät] - [Fachbereich Chemie] - [Anorg. Chemie] - [Klaus Eichele] - [NMR Ramblings] - [Processing] - [Convolution] - Shifted Sine Bell - SINE, QSINE
 |
NMR Processing: |
Function:
The figure shows the continuous example FID after sinm with SSB = 1, SSB = 2, and SSB = 8;
these are datasets fid/104, fid/105, and fid/106 in the download package.
Sine-bell multiplication multiplies the FID with a sinusoidal function with a period twice the acqusition time. The value of SSB determines the phase of the
sinusoidal function and results in the following filter functions:
| SSB | Result |
| 0, 1 | sine |
| 2 | cosine |
| >2 | increasingly sine |
Examples/Exercises:
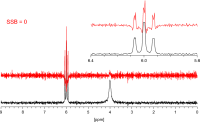 |
Click on the figure to see how sinm with SSB = 0 or SSB = 1 (sine) applied to the synthetic dataset synth/112 in the download package affects the spectrum. Note the resolution enhancement and apodisation at the expense of signal-to-noise ratio. Also, note the negative contributions. |
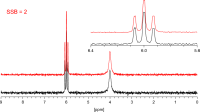 |
Click on the figure to see how sinm with SSB = 2 (cosine) applied to the synthetic dataset synth/113 in the download package affects the spectrum. Note the apodisation and slight increase of signal-to-noise ratio without significant loss in resolution. |
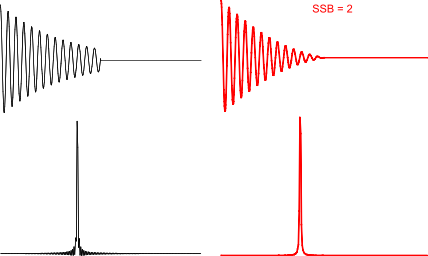 |
The apodization effect is also visible in this second example: A truncated FID shows after zero-filling and FT excessive "wiggles" in the spectrum (black, left). Application of a cosine filter results in apodization without significant broadening (red, right). |
| SSB > 2: weighted mixture of both objectives. |
Properties/Purpose:
- the resolution enhancement is only modest
- may result in negative wings of the peaks -> integration is hampered
- primarily used for 2D spectra, especially if the acquisition time is short and the FIDs truncated
- also used for 2D spectra to convert Lorentzian line shapes into something with less excessive wings; to that end, the squared sine bell version might be more useful
Literature:
- Hoffman, Levy, Progr. NMR Spectrosc. 1991, 23, 211; doi:10.1016/0079-6565(91)80005-M
- Lindon, Ferrige, Progr. NMR Spectrosc. 1980, 14, 27; doi:10.1016/0079-6565(80)80002-1
- Gassner, Jardetzky, Conover, J. Magn. Reson. 1978, 30, 141; doi:10.1016/0022-2364(78)90234-2
- Guéron, J. Magn. Reson. 1978, 30, 515; doi:10.1016/0022-2364(78)90277-9
[ Anorg. Chemie ] | [ Go Home ] | webm@ster | last modified: 18.03.2020

